The Complete Evidence that Starling’s Law is Wrong: The Correct Replacement is the Hydrodynamic Phenomenon of the Porous Orifice (G) Tube

Introduction and objective: To report the hydrodynamic of a porous orifice (G) tube as replacement for the wrong Starling’s law.

Material and methods: Hydrodynamics of the G tube, based on capillary ultra-structure, were studied. The effect of changing G tube orifice diameter, proximal pressure and distal pressure on the side pressure and chamber (C) pressure were evaluated. The physiological proof that the capillary works as G tube not Poiseuille’s tube is provided.

Results: Hydrodynamics of the G tube showed that proximal, akin to arterial, pressure induces a negative side pressure gradient on the G tube wall, which is negative causing suction maximum near the inlet and turn positive near the exit causing filtration. This created the rapid, autonomous magnetic field like fluid circulation phenomenon between G and C. The physiological evidence on the hind limb of sheep proves that the capillary works as G tube.

Conclusion: Hydrodynamic of the G tube challenges the role attributed to arterial pressure as a filtration force in Starling’s law. A literature review shows that oncotic pressure does not work and the law has failed to explain the capillary-ISF transfer. A concept based on the new hydrodynamic phenomenon of the G tube is proposed to replace Starling’s law. A rapid autonomous dynamic magnetic field-like G–C circulation occurs. Factors which initiate, regulate and affect the G–C circulation, its physiological proof and relevance to clinical importance are given. A physiological evidence on capillary working as G tube not Poiseuille’s tube is provided.

VO: Volumetric Overload; VOS: Volumetric Overload Shocks; VOS1: Volumetric Overload Shock, Type 1; VOS2: Volumetric Overload Shock, Type2; TURS: The Transurethral Resection of the Prostate Syndrome; ARDS: The Adult Respiratory Distress Syndrome; MVOD/F: The Multiple Vital Organ Dysfunction/Failure Syndrome; HN: Hyponatraemia; BP: Arterial Blood Pressure; CVP: Central Venous Pressure; ISF: Interstitial Fluid Volume; G Tube: The Porous Orifice Tube; PP: Proximal Pressure to the G tube akin to arterial Blood pressure; DP: Distal Pressure to the G tube akin to venous pressure; LP: Lumen Pressure of the G tube; FP: Flow Pressure is the positive pressure inside the G Tube; SP: Side Pressure is the negative pressure inside the G Tube.

In 1886, Starling proposed a hypothesis for the capillary-Interstitial Fluid (ISF) transfer, in which the capillary was thought a tube of a uniform diameter and its wall is impermeable to plasma proteins [1]. The flow of fluid across its wall was thought dependent upon a balance between the hydrostatic pressure within its lumen (LP), encouraging fluid to leave ‘filtration’, and the osmotic pressure of plasma proteins or oncotic pressure (pc), tending to draw fluid back to the capillary lumen ‘absorption’, with similar minor opposing forces in the ISF space. At the arterial end of the capillary, Lumen Pressure (LP) is greater than the oncotic pressure and fluid is pushed out. At the venous end, oncotic pressure is greater than LP and fluid is drawn in. The physics basis on which LP of a capillary was thought positive and responsible for filtration was Poiseuille’s work (1799–1869) on long Brass tubes of uniform diameters [2]. However, Burnoulli’s effect of a fluid jet and Venturi’s effect of a tube constriction are well known and must also be considered to be of significance even under laminal flow conditions. Starling’s hypothesis has undergone extensive evolution to become a law prior to the discovery of the capillary ultra-structure of the pre-capillary sphincter [3] and its porous wall [4] plus the osmotic chemical composition [5], negative pressure of ISF [6] and lymph [7]. Inadequacy in explaining the capillary-ISF transfer in many parts of the body [8], particularly vital organs, has previously called for reconsideration of Starling’s hypothesis [9]. In 1984, clinical observations inconsistent with Starling’s hypothesis prompted a physical study to verify LP and fluid dynamics in a porous orifice tube with reference to the effect of the pre-capillary sphincter, arterial and venous pressures on the capillary-ISF transfer. The observation was: during hypotension shock in which prompt and adequate vascular expansion for resuscitation, all fluids leaked into and drowned the ISF space and filled the potential body cavities, demonstrated on post-mortem examination [10-12].

Folkow and Neil [2] stated that: ‘The greatest discovery in medical science was made by William Harvey, who showed in 1628 that the heart pumped the blood around the circulatory system and understood that the circulation provided nourishment for the tissues’. Four centuries earlier, however, Ebn Al-Nafis (1210–1298), discovered the pulmonary circulation. Both the systemic and pulmonary circulations exist to provide for tissues’ viability. Capillary circulation is directly responsible for this vital function in every organ and tissue. In 1886, Starling [1], a great physiologist, proposed a hypothesis for the capillary-ISF exchange. It was based on two known physical forces; LP based on Poiseuille’s tube as a filtration force and the oncotic pressure as a absorption force. In 1929, Landis [13] measured LP of a capillary, by a cannula facing up stream, which was 32 and 12 mmHg at the arterial and venous ends, respectively. In 1948, Pappenheimer and Soto-Rivera [14] studied the quantitative changes in the weight of isolated hind limbs of dogs induced by alterations of the arterial and venous pressures. Oedema was induced by increasing the venous pressure in order to match any increase in the arterial pressure (mmHg for mmHg). Such, ‘isogravimetric state’, in which capillary filtration exceeds absorption, as would be expected with high venous pressure, was thought in support of Starling’s hypothesis. The authors also found that the osmotic pressure of a concentrated plasma solution was 23–28 mmHg in vitro. Ever since, most physiologists have accepted Starling’s hypothesis as a physiological law. However, most of the enlightening scientific evidence on microcirculation started to appear decades later. In 1960, Mellander [15] showed that ISF ‘absorption’ increased after autonomic stimulation, which narrows the lumen of ‘micro-vessels’ and increases arterial pressure. In 1962, Hendry [5] measured the oncotic pressure of various body fluids and found it identical to that of the plasma, pointing out: ‘the osmotic pressure of plasma proteins is too weak and too slow force to return fluids back into the capillary lumen’. In 1963, Guyton and Colman [6] measured ISF tissue pressure, using a subcutaneously implanted perforated capsule, and found that it has a negative value of –7 cm water. In 1972, Calnan et al. [7] confirmed this finding and showed that molecules, including plasma proteins, pass freely and rapidly between the capillary blood and the implanted capsule, and vice versa. In 1967, Rhodin [3] showed that the capillary tube is encircled by a cuff of smooth muscle fibres at its arteriolar junction, named the pre-capillary sphincter (3–5 µm), which is the narrowest part of the whole vascular system. Also in 1967, Karnovesky [4] showed that the capillary wall is made of flat cells and their intercellular junctions are slits 10 to 20 nm wide which are the pores through which fluids, nutrients and protein molecules pass freely. His photographs show the stained horse radish globules, which are much larger than plasma protein molecules, passing through these pores. The findings, concerning plasma proteins and capillary pores alone, have cancelled the absorption force in Starling’s law. In 1983, Mattfeldt and Mall [15] reported the ultra-structure dimensions of capillaries. The ‘ideal’ capillary is a tube connecting an arteriole to a venule. According to Crogh’s model it is a perfect, anisotropic, straight and un-branched tube with a diameter of (7–18 µm). The pre-capillary sphincter and intercellular slits make the capillary a strait ‘porous orifice tube’, based on which the G tube was made on a larger scale. In 1982, Keele, Neil and Joels [8], pointed out that the concentration of tissue proteins in the liver, lung and muscles is 60% that of plasma proteins. In the pulmonary circulation the arterial pressure is less than the plasma oncotic pressure. Thus fluid filtration in the lungs and absorption in the liver and muscles lacks explanation. The oncotic pressure neither can explain the negative pressure in ISF space [6] nor the speed and efficiency with which the capillary–ISF fluid, nutrients and oxygen transfer occurs. Lymph drainage cannot explain the negative ISF pressure [6,7]. Neurohormonal stimulation enhances absorption [16]. This knowledge on capillary ultra-structure and permeability to macromolecules, prompted Renkin (1986) to call for reconsideration of Starling’s hypothesis [9] but an alternative mechanism was evolving at this time. This investigation was preliminary reported [10].

In Poiseuille’s tube with a uniform diameter fluid exerts a positive SP gradient on its wall, causing fluid to leak out through holes all along its wall. In an orifice tube, however, fluid exerts a negative SP gradient, causing fluid to be drawn in by suction from the outside which is maximal over the proximal part near the inlet or ‘arterial’ end (Figure 1).

SP turns positive at the distal part near the exit (Figure 2). Using the G tube partially immersed in water, fluid flows out from side holes near the outlet or the ‘venous’ end while it flows in by suction through holes near the inlet or ‘arterial’ end, re-entering back into the lumen of the G tube. Fluid around the G tube moves in an opposite direction to flow inside its lumen in a magnetic field-like pattern (Figure 2 and 3).

The inflow PP and orifice induce a fluid jet. LP of a fluid jet has 2 components: FP is a positive energy LP component responsible for the flow. SP is a negative energy pressure gradient responsible for suction. Both suction and filtration are autonomous effects of a flow in the G tube. In the G–C model, a rapid mixing autonomous circulation occurs between fluid in the lumen of the G tube and chamber C compartments (Figure 3).

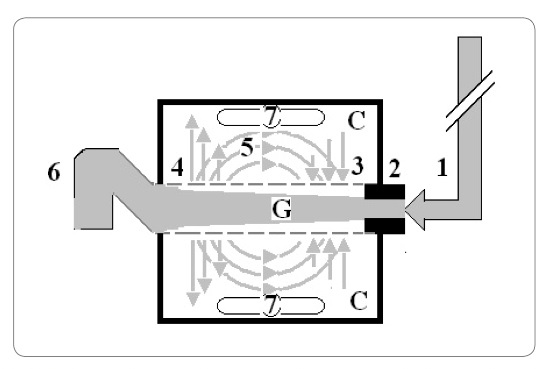
Figure 3: Shows diagram of the porous orifice (G) tube enclosed in chamber (C) based on several photographs demonstrating the magnetic field-like G-C circulation phenomenon. The proximal inflow (arterial) pressure (1) pushes fluid through the orifice (2) creating fluid jet in the lumen of the G tube. The fluid jet creates negative side pressure gradient causing suction maximal over the proximal half of the G tube near the inlet (3) that sucks fluid into lumen. The side pressure gradient turns positive pushing fluid out of lumen over the distal half maximally near the outlet (4). Thus the fluid around G tube inside C moves in magnetic field-like fluid circulation (5) taking an opposite direction to lumen flow of G. tube. The inflow (arterial) pressure (1) and orifice (2) induce the negative side pressure energy creating the dynamic G-C circulation phenomenon that is rapid, autonomous and efficient in moving fluid out from the G tube lumen at (4), irrigating C at (5), then sucking it back again at (3), maintaining net negative energy pressure (7) inside C. The distal outflow (venous) pressure (6) enhances outflow at (4) and its elevation may turn the negative energy pressure (7) inside C into positive, increasing volume and pressure inside C chamber. 

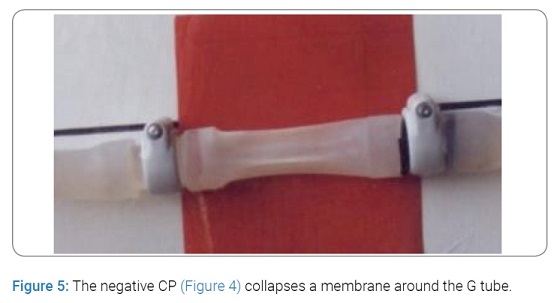
This G–C circulation phenomenon also induces a net negative energy in chamber (CP), demonstrated in (Figure 4 and 5).

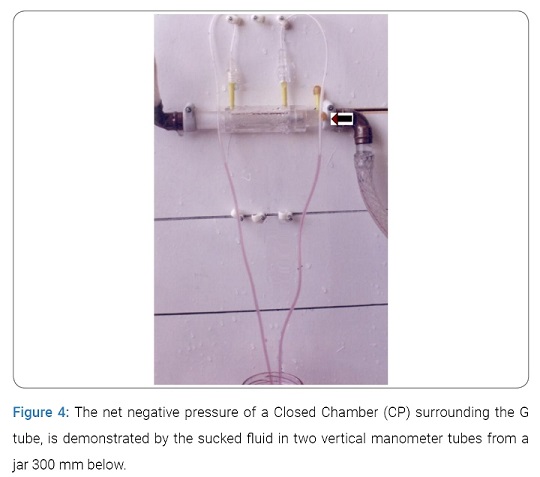
A pressure gradient also occurs inside chamber C, causing fluid to move in an opposite direction to the main flow in the G tube lumen.

The inflow PP, orifice/tube ratio and the outflow DP interact to regulate pressure gradient of the G tube, the speed of the G-C circulation and fluid volume and CP in chamber C. Increasing PP augments the negative SP and CP, increases the speed and efficiency of the G–C circulation, and vice versa. Increasing DP augments filtration, counteracted suction, slowed down the G–C circulation and increased fluid volume and CP in chamber C. The dynamic autonomous magnetic field-like G–C circulation phenomenon, its pressure gradient and relation to PP, orifice and DP are demonstrated in a circulatory system model (Figure 6-8).

The G–C circulation is so fast in mixing fluid inside the G tube and chamber C that it cleared any injected ink into chamber C as soon as the injection ceased (Figure 8).

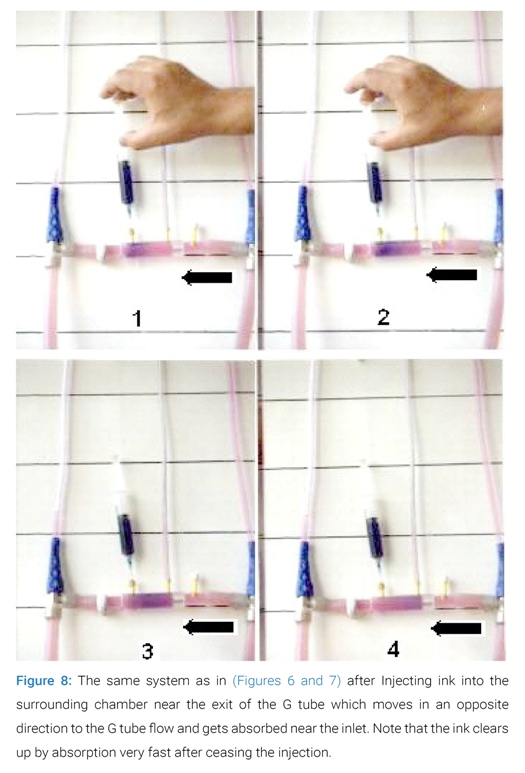
Fluid and particles move freely between the G tube lumen and chamber C, limited only by pore diameter and flow kinetics. Reproducible results were obtained in thousands of experiments done under steady PP head and using a circulatory system model. The physiological and clinical relevance of results have been discussed with reference to its physiological equivalents outlined in the reviewed literature as well as known pathological conditions and mentioned clinical observations [10]. The effect of orifice diameter on SP and CP are shown in (Figure 9).

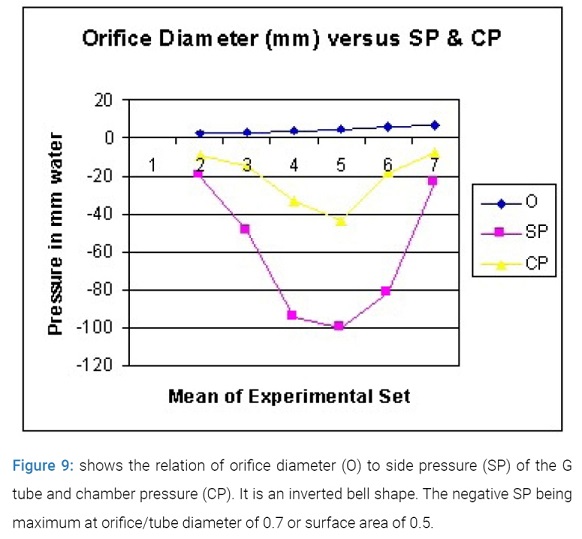
It is bell shape being maximum at orifice of 0.7 of tube diameter, which is 0.5 the surface area. The relation of DP to SP and CP is shown in (Figure 10).

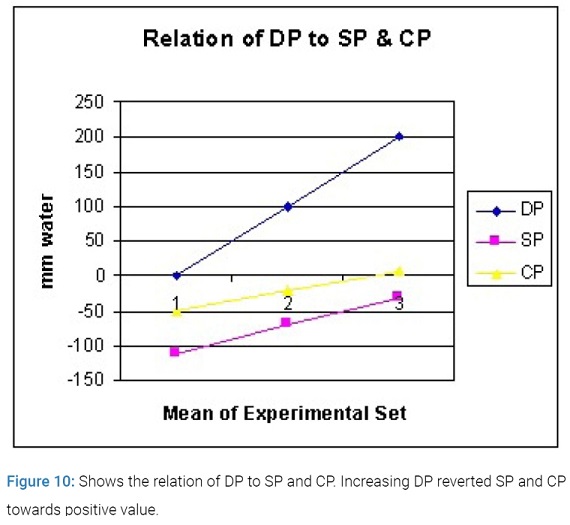
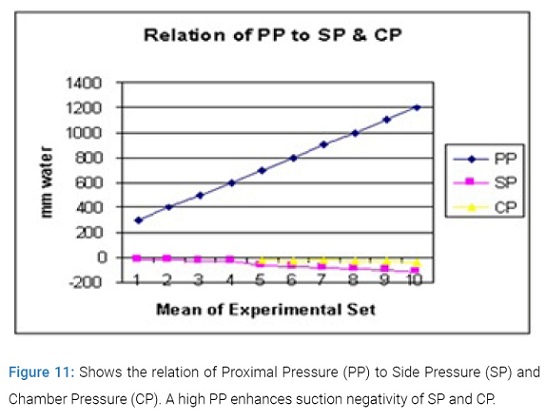
Increasing DP causes elevation of SP and CP towards positive. (Figure 11), shows the relation of PP to SP and CP. A high PP enhances suction negativity of SP and CP and augments the G-C circulation.

Results demonstrate that the hydrodynamics of the G tube are different from that of Hagen (1839)–Poiseuille’s tube (1840), which in turn challenge the role attributed to arterial pressure as the filtration force in the capillary circulation as proposed by Starling [1]. Inflow PP induces a fluid jet with LP inside both the G tube and Poiseuille’s tubes, which has the dynamic FP and SP components. Over the proximal part of the G tube, the net effect of the negative energy SP gradient is suction (Figure 1), but in Poiseuille’s tube it is filtration. Both FP and SP components of a fluid jet are extensions of the well known Bernoulli (1738) and Venturi effects. The major difference between Poiseuille’s and the G tubes, is that the first induces an all positive energy pressure gradient which can only cause filtration along the entire length of a tube – hence it requires another force for absorption. The G tube has both its positive and negative energy pressure components which autonomously induce both filtration (Figure 2) and absorption (Figure 1), inducing the G–C circulation phenomenon (Figure 3) and the net negative CP in a surrounding fluid chamber C (Figure 4,5). Physiological relevance and clinical significance of the G–C phenomenon are discussed here.

Pitfalls of Starling’s Hypothesis: The physics and clinical investigations were previously reported [10-12]. Dr Starling proposed his hypothesis 80 years prior to the discovery of the pre-capillary sphincter [3]. The hypothesis has failed to explain the capillary–ISF transfer in most parts of the body [8]. For the oncotic pressure to work an impermeable membrane to plasma proteins must exist but it does not. For the capillary to work on the basis of a positive pressure gradient of Poiseuille’s tube, which causes filtration all along the tube, another force must exist for re-absorption, and there is none. It neither explains Guyton and Colman’s discovery of the negative ISF pressure [6] nor how venous obstruction or pressure elevation causes oedema while arterial hypertension never does. The measured LP of the capillary tube by Landis [13] is akin to measuring FP in the G tube. At that time, there was no distinction between the components of a dynamic flow, as presented here. LP of the capillary was thought responsible for filtration and its elevation was thought possible by elevating venous or arterial pressures alike, as demonstrated by the isogravimetric experiments done by Pappenheimer and Soto-Rivera [14]. Received thinking that elevating CVP is synonymous with elevating arterial pressure is prevailing in current clinical practice during fluid therapy for shock and the management of the acutely ill patients [14]. This is undoubtedly correct during restoration therapy for hypovolaemic and haemorrhagic shock, but vascular expansion or VO is a different issue. Persistent attempts to elevate CVP and PCWP up to levels of 18 to 22 cm water are common received practice. The normal CVP, however, is around 0 and most textbooks report a range of –7 to +7 cm water [17,18]. The pointed out clinical observations demonstrate that, in addition to the well known effect of high venous pressure causing oedema, arterial hypertension has no such effect, if not the exact opposite. In clinical practice, although arterial hypertension is common, ISF oedema is unknown among its complications. In the G–C model, a minor increase in DP increases fluid volume in chamber C, reverts its CP from negative to positive while slowing the G–C circulation (Figure 6,7 and 10). Increasing DP has similar effects to decreasing PP on the G–C circulation and chamber pressure and volume. Vascular expansion causes VO shocks [19-21]. A physiological study [22] was conducted n the hind limb of sheep brought from the Butcher shop and wrapped in a cling film to replace the skin. Both normal saline and plasma proteins were used as circulatory fluid. An electric pump was used to circulate fluid once through the main artery and compared it to another running fluid through the main vein. The formation of oedema was observed by the increase in the limp weight and accumulation of fluid under the cling film. Here is the abstract of this physiological study.

Hydrodynamic studies on a porous orifice (G) tube, based on capillary ultra-structure, demonstrate results which differ from Poiseuille’s in a strait uniform tube and hence challenge the role attributed to arterial pressure as a filtration force in Starling’s law. A perspective literature review shows that the oncotic pressure force has been previously cancelled and the law has failed to explain the capillary–ISF transfer in most parts of the body. A concept based on a new hydrodynamic phenomenon is proposed for the capillary–ISF circulation. It explains this vital circulation in every organ and tissue under both physiological and pathological conditions. A rapid autonomous dynamic magnetic field-like G–C circulation occurs between fluid in the G tube’s lumen and a surrounding fluid compartment C. Based on results of studies on a circulatory model incorporating the G–C apparatus, factors which initiate, regulate and affect the G–C circulation, its physiological and haemodynamic relevance and its clinical importance to the pathogenesis of oedema, shock and the MVOD/F syndrome are outlined. The presented evidence does not only prove that Starling’s law is wrong, but also provide the correct replacement; the hydrodynamic of the G tube explaining the capillary-ISF circulation. Also the physiological evidence on capillary working as G tube not Poiseuille’s tube is provided. The presented evidence proves without a doubt that Starling’s law is wrong and provide its replacement of the G tube dynamics.

I thank Professors GD Chisholm, Eric Neil, Dr Antony Winward, Mr BJ Stoodley and Mr PL Brooks for helpful comments and encouragement, Mr Peter Holder, Designer Engineer, Eastbourne, for making the G–C model and board used for quantitative measurements and Mr and Mrs Freda and Robert Prentice for permitting the generous use of their premises at 70, Glendale Avenue, Eastbourne, BN21 UN, during the G tube studies.

1. Starling EH. Factors involved in the causation of dropsy. Lancet. 1886;1266–70.
2. Folkow B, Neil E. Circulation. Oxford University Press: London. 1971;1–125.
3. Rhodin JA. The ultra structure of mammalian arterioles and precapillary sphincters. J Ultrastruct Res. 1967;18(1):181–223.
4. Karnovesky MJ. The ultra structural basis of capillary permeability studied with peroxidase as a tracer. J Cell Biol. 1967;35(1):213–36.
5. Hendry EB. The osmotic pressure and chemical composition of human body fluids. Clin Chem. 1962;8:246–65.
6. Guyton AC, Coleman TG. Regulation on interstitial fluid volume and pressure. Ann N Y Acad Sci. 1968;150(3):537–47.
7. Calnan JS, Pflug JJ, Chisholm GD, Taylor LM. Lymphatic surgery. Proceedings Royal Soc Med. 1972;65:715–9.
8. Keele CA, Neil E, Joels N. Sampson Wright Applied Physiology. 13th ed. Oxford University Press; Oxford. 1982.
9. Renkin EM. Some consequences of capillary permeability to macromolecules: Starling’s hypothesis reconsidered. Am J Physiol. 1986;250(5 Pt 2):H706–10.
10. Ghanem AN. Magnetic field-like fluid circulation of a porous orifice tube and its relevance to the capillary-interstitial fluid circulation: preliminary report. Med Hypotheses. 2001;56(3):325–34.
11. Ghanem AN. The Transurethral Prostatectomy (TURP) Syndrome: An Investigation of the Osmotic and Metabolic Sequelae of Volumetric Overload. MD Thesis. Institute of Urology and Nephrology, Mansoura University, Mansoura, Egypt. 1988.
12. Ghanem AN1, Ward JP. Osmotic and metabolic sequelae of volumetric overload in relation to the TUR syndrome. Br J Urol. 1990;66(1):71–8.
13. Landis EM. Micro-injection studies of capillary blood pressure in human skin. Heart. 1929;31(15):209–28.
14. Pappenheimer JR, Soto-Rivera. Effective osmotic pressure of plasma proteins and other quantities associated with capillary circulation in the hind limbs of cats and dogs. Am J Physiol. 1948;152(3):471–91.
15. Mattfeldt T, Mall G. Estimation of length and surface of anisotropic capillaries. J Microsc. 1984;135(2):181–90.
16. Mellander S. Comparative studies on the adrenergic neurohormonal control of resistance and capacitance blood vessels in the cat. Acta Physiol Scand Suppl. 1960;50(176):1–86.
17. Webster NR. Monitoring the critically ill patient. J R Coll Surg Edinb. 1999;44(6):386–93.
18. Guyton AC. Textbook of Medical Physiology. An HBJ International Seventh Edn. WB Saunders Company. Philadelphia London. 1986;19:221.
19. Ghanem AN, Ghanem, SA. Volumetric Overload Shocks: Why Is Starling’s Law for Capillary Interstitial Fluid Transfer Wrong? The Hydrodynamics of a Porous Orifice Tube as Alternative. Surgical Science. 2016;7:245–9. 
20. Ghanem SA, Ghanem KA, Ghanem AN. Volumetric Overload Shocks in the Patho-Etiology of the Transurethral Resection of the Prostate (TURP) Syndrome and Acute Dilution Hyponatraemia: The Clinical Evidence Based on Prospective Clinical Study of 100 Consecutive TURP Patients. Surg Med Open Access J. 2017:1(1);1–7.
21. Ghanem KA, Ghanem AN. Volumetric overload shocks in the patho-etiology of the transurethral resection prostatectomy syndrome and acute dilution hyponatraemia: The clinical evidence based on 23 case series. Basic Res J Med Clin Sci. 2017;6(4):35–43.
22. Ghanem KA, Ghanem AN. The Physiological Proof that Starling’s Law for the Capillary-Interstitial Fluid Transfer is Wrong: Advancing the Porous Orifice (G) Tube Phenomenon as Replacement. Open Acc Res Anatomy. 2017;1(2):OARA.000508.

Capillary Physiology; Hydrodynamics; Starling’s Law; Fluid Therapy; Shock; Hyponatramia; Acute Respiratory Distress Syndrome (ARDS)

Ahmed N Ghanem. The complete evidence that starling’s law is wrong: the correct replacement is the hydrodynamic phenomenon of the porous orifice (g) tube. Clin Surg J. 2020;1(1):1–7.

© 2020 Ahmed N Ghanem. This is an open access article distributed under the terms of the Creative Commons Attribution 4.0 International License (CC BY-4.0).